The coordinated El Niño/Southern Oscillation
phenomenon (ENSO, for short) is the strongest source of natural variability
in the global climate system [41].
During the severe ENSO event 1982/1983, when the sea surface off Peru warmed
by more than 7° C, it was discovered that there are strong links to
weather in other regions as, for instance, floods in California and intensified
drought in Africa. The observation of this global connection implied that
the oceanic and atmospheric anomalies of the equatorial Pacific might be
the key to accurate seasonal weather forecasts in other regions. Since
then there has been a continuous search for ENSO precursors and worldwide
patterns of effect to make it possible to predict the event and its consequences
for seasonal weather. This effort has a solid basis as far as the involved
energy is concerned. J.
L. Daly [9] has
shown, that positive and negative anomalies in the global temperature of
the lower troposphere, measured by satellites since 1979, are primarily
driven by El Niños, La Niñas, and the Southern Oscillation.
This is not easy to see as global temperature lags the SOI by 6 to 9 months.
Only when severe volcanic eruptions occur, global temperature is modulated
by their cooling effect.
J. P. Peixoto and A. H. Oort [40]
have provided similar evidence covering the period 1958 - 1987. Their
data include the whole troposphere and the lower stratosphere up to 25
km height. When they compared the time series of SST anomalies in the eastern
equatorial Pacific with the time series of the atmospheric temperature
averaged vertically and horizontally over the entire Northern Hemisphere,
they found that the curves are highly correlated. The correlation is highest
( r = 0.82) when the atmospheric temperature lags the ocean temperature
by four months. They concluded: "Since correlations
with the mean Southern Hemisphere temperatures are also very high, it is
clear that a large part of the observed variability in the global atmosphere
must be connected with the ENSO events." Peixoto and Oort
[40] also observed
that sea surface temperatures lag the SOI by 4.5 months. So they found
that atmospheric temperatures lag the SOI by 8.5 months. This is just what
Daly found in the satellite measurements of the lower troposphere.
When we look at Figure 1 and the rather irregular
oscillations in the plotted time series, it seems impossible to predict
ENSO events on a theoretical basis. Yet the NOAA tripwire open-ocean buoy
array including 65 deep-ocean moorings and 135 surface drifters gives climatologists
an early warning of 6 to12 months of an impending El Niño. In addition,
a satellite borne microwave sensor allowed scientists in 1997/1998 to estimate
the velocity and track of the large eastward-travelling Indonesian Kelvin
wave and the quantity and temperature of the hot water migration from west
to east. Daily observations of changes in SST, surface wind, upper ocean
thermal structure, and ocean currents enable researchers to engage in modeling
and predicting ENSO events. It seems to be very difficult, however, to
develop models that extend the 1-year limit set by the observation of precursors.
M. Cane and S. Zebiak of the Lamont-Doherty Earth Observatory of Columbia
University made the first successful forecast of an El Niño in early
1986, one year ahead of the event, but their model did not indicate the
strong 1997/1998 El Niño. At present, there exist no models that
can skillfully predict ENSO events at lead times longer than twelve months
[36].
Neelin and Latif [36]
think that ENSOís irregularity limits its predictability. They hold
that weather noise, the changing background state of climate, and deterministic
chaos, representing the internal variability of the climate system, set
the fundamental limits to the lead time at which El Niño can be
predicted. This view that ENSO events are exclusively internal phenomena
of the climate system represents the accepted teaching in climatology,
as expressed by Peixoto and Oort [40]:
"On the interannual
time scale there are no large external forcings of the atmosphere-ocean
system so that the variations must arise from internal interactions with
many positive and negative feedbacks. The most spectacular example of an
internal variation is the ENSO phenomenon that may be regarded as a free
oscillation of the ocean-atmosphere system."
I shall show that this is a preconception.
Actually, El Niño and La Niña are subjected to external forcing
by the sunís varying activity to such a degree that it explains nearly
all of ENSOís irregularities and makes long-range forecasts beyond the
1-year limit possible. This is no mere theory. My forecasts of the last
two El Niños [28]
turned out correct and that of the last one was made more than two
years ahead of the event, beyond the 1-year limit discussed in the literature.
Deterministic chaos, mentioned by Neelin and Latif, does not stand in the
way of external forcing. Sensitive dependence on initial conditions and
ensuing limited predictability are only valid with regard to processes
within the climate system. External periodic or quasiperiodic systems can
positively force their rhythm on it. This is not only the case with the
periodic change of day and night and the Milankovitch cycles, but also
with variations in the solar energy output as far as they are periodic
or quasiperiodic.
 2.
11-year sunspot cycle and the Golden section
2.
11-year sunspot cycle and the Golden section
The 11-year sunspot cycle meets these conditions
of external forcing. Yet climatologists who exclusively consider the change
in the sunís irradiance solely look at maxima and minima of the sunspot
cycle. It is easy to see that these extrema show no consistent and sufficiently
strong correlation with the El Nino phenomenon. However, recent research
has shown that the solar wind, driven by solar eruptions (flares and coronal
mass ejections) and plasma flux emanating from coronal holes, has a stronger
impact on climate phenomena either directly or by modulating galactic cosmic
rays [31]. The
activity of coronal holes is not well correlated with sunspot activity,
while energetic eruptions shun sunspot maxima and occur even close to minima.
So it makes sense to investigate whether there are other phases than maxima
and minima within the sunspot cycle which are closely correlated with El
Niño events.
The 11-year sunspot cycle is not built symmetrically.
The ascending part from minimum to maximum is shorter than the declining
part from maximum to minimum. I have shown that the sunspot maximum divides
the sunspot cycle according to the Golden section. It falls at the minor
of this special irrational proportion. The Golden section divides a frame
structure like a line segment, a surface, a cycle, or any other delimited
feature so that the ratio of the smaller part (minor) to the larger part
(major) equals the ratio of the larger part to the whole. When we set the
whole equal to 1, we get 0.3819 ... : 0.618 ... = 0.618 ... : 1. To find
the major of the length of a cycle, it has to be multiplied by 0.618. Multiplication
by 0.382 gives the minor.
Reliable sunspot data are available since
1750. They show that the ascending part of the sunspot cycle has a mean
length of 4.3 years. The mean cycle length amounts to 11.05 years. The
minor of the mean length falls at 4.2 years (11.05 yrs *
0.382 = 4.22 yrs). This is close to 4.3 years, the observed mean of the
interval from minimum to maximum. The descending part of the cycle has
the length of the major. Magnetic cycles of solar type stars show the same
structure shaped by the Golden section [28].
This is not merely a queer coincidence. The Golden section has a physical
function. A. N. Kolmogorov [17],
V. I. Arnolíd [1],
and J. Moser [34]
have proven theoretically that the stability of the solar system hinges
on the Golden section. This is crucial, as we know from publications by
G. J. Sussman and J. Wisdom [51]
and also J. Laskar [32] that the
orbits of all planets are chaotic. In my paper "The
Cosmic Function of the Golden Section" [28]
I have shown how the Golden section, which stands for stability in
polar opposition to instability, has kept the solar system stable for 4.6
billion years in spite of chaos in all planetary orbits. The circumstance
that the sunspot maximum falls at the minor of the sunspot cycle contributes
to the stabilization of solar activity which is characterized by phenomena
generated by instability. The sunspot cycle is no isolated case. It confirms
the rule that minor and major of the Golden section within solar-terrestrial
cycles indicate special phases with outstanding effects. I refer to numerous
examples given in my paper "Solar
Activity: A Dominant Factor in Climate Dynamics" [31].
Thus, an investigation of a potential correlation between the sunspot
cycle and El Niños should take this point into consideration.
 3.
Fractal character of the sunspot cycle and energetic solar eruptions
3.
Fractal character of the sunspot cycle and energetic solar eruptions
Nature is fond of fractals. It makes use
of well-tried patterns on different scales. The ascending and the declining
part of the sunspot cycle could be looked at as cycles in their own right.
So it should be considered whether these smaller cycles repeat the pattern
of the whole sunspot cycle such that the minor of the Golden section indicates
a phase of outstanding activity. To test this hypothesis I checked the
distribution of energetic solar eruptions within the two subcycles. This
is together the involvement of a phenomenon that drives the solar wind.
I chose all X-ray eruptions equal to or greater than X6, observed by satellites
from 1970 to 1998 [35].
Intense X-ray flares have a stronger impact on climate than flares categorized
into classes of optical brightness [25].

Figure 2 shows the superposed result
for both of the subcycles which are normalized to emphasize identical phases
in cycles of different length. The phase of the minor 0.382 is marked by
a solid triangle. The energetic flares concentrate on the interval between
0.225 and 0.45 around the minor. As much as 19 of the 34 observed energetic
flares fall at this interval and only 15 at the remaining interval covering
a range of 0.775 on the unit scale. A chi-square test yields the value
21.7 for 1 degree of freedom. The probability that the observed distribution
is due to chance is P = 0.000003. The sceptical null hypothesis can be
considered rejected.
 4.
Distribution of El Niños within subcycles of the sunspot cycle since
1610
4.
Distribution of El Niños within subcycles of the sunspot cycle since
1610
So it seems to make sense to look for a potential
relationship between special phases in the ascending and declining subcycles
of the 11-year sunspot cycle and El Niño events. Results based on
short time series are always exposed to objections. This is why data covering
nearly four centuries were chosen. Epochs of sunspot minima and maxima
are available since 1610. El Niño data published by W. A. Arntz
and E. Fahrbach [2],
partly based on W. H. Quinn, V. T. Neal, and S. E. A. de Mayola [43],
even go back beyond 1610. Only years of the event or periods of two years
are given. To proceed consistently, the middle of single years, or the
beginning of the second year of pairs of years was assumed to give the
closest approximation to the event date. The dates of El Niños before
1875 and of sunspot extrema before 1750 cannot be considered precise, but
if there is a strong correlation, it should nevertheless emerge. Figure
3 shows the result.

There is the expected concentration of events
around the minor 0.382 of the Golden section. 19 El Niños of the
total of 60 fall at the interval between 0.32 and 0.5 and only 41 at the
remaining interval 0.82. A chi-square test yields the chi-square value
7.6 and P = 0.006. The sceptical null hypothesis can be rejected at a high
level of significance. Yet there are other deviations from the expected
distribution. The accumulation around the major 0.618 complements the Golden
section phases. This is also true of the concentrations around 0.146 and
0.854 though this is not easy to see. Practice shows that the larger intervals
within a cycle between the initial phase and the minor and between the
major and the following initial phase are often bridged by Golden sections
of the second generation. The additional phase 0.146 falls at the minor
of the interval between 0 and the minor 0.382, while the further phase
0.854 coincides with the major in the interval between the major 0.618
and 1. The phases 0.146, 0.382, 0.618, and 0.854 are symmetrically distributed
about the phase 0.5 in the middle of the cycle. The accumulation at the
phase 0, representing sunspot maxima and minima, is a surprise, as recent
data show no connection of this kind. A statistical evaluation with the
ranges of accumulation as indicated in Figure 3 by delimited horizontal
lines yields a chi-square value 44.4 for 1 degree of freedom and P = 3×10
-11. This clearly indicates that
we are not dealing with a chance distribution, though there was no starting
hypothesis covering the outstanding phases 0.146 and 0.854.
 5.
Distribution of SOI extrema within subcycles of the sunspot cycle since
1951
5.
Distribution of SOI extrema within subcycles of the sunspot cycle since
1951
These first results should be checked by
data that are not affected by inaccuracies and come as close as possible
to the cause of ENSO events. Such events are characterized by a low negative
Southern Oscillation Index (SOI), weaker than normal trade winds over the
central Pacific, and warmer than normal sea surface temperatures (SST)
in the eastern equatorial Pacific. A low SOI goes along with a reduced
westward pressure gradient and changing wind stress values. According to
Peixoto and Oort [40]
the pressure change comes first, whereas the wind stress lags the SOI
by two months. The SST lags it even by 4.5 months. So variations in the
pressure gradient, expressed as change in the SOI, are closer to the cause
of ENSO events than wind stress and rising sea surface temperature. This
is why I investigated standardized monthly data of the SOI covering the
period 1951 - 1998 [38].
The curve in Figure 4 shows these
slightly smoothed data.

They have been inverted so that they run
parallel with El Niños and La Niñas. The vertical scale on
the right indicates the original orientation. Future verbal references
to the SOI will always refer to this original orientation linked to the
right scale. Obviously, extrema in the SOI are closely correlated with
Golden section phases within the ascending (a) and declining (d) part of
the 11-year sunspot cycle which already emerged in the El Niño investigation
covering four centries. They are indicated by yellow and blue triangles.
The minor 0.382 (yellow) with the strongest correlation in the earlier
analysis appears as well in the ascending subcycle (0.382 a) as in the
descending subcycle (0.382 d). After the year 1968, designated by arrows,
the minor (0.382 a,d) marks precisely and without exception all observed
strong negative extrema in the SOI, which are at the same time strong El
Niños. Before 1968 the minor coincides with positive extrema in
the SOI, which are at the same time La Niñas. The second generation
phase 0.854 (blue) emerges only in the declining subcycle (0.854 d). Before
1968 it is consistently correlated with El Niños and after 1968
with La Niñas. The pattern is reversed in relation to the distribution
of the minor 0.382. The overall impression is that of a symmetric distribution.
 6.
Phase reversals in climate cycles due to a fractal of solar cycles
6.
Phase reversals in climate cycles due to a fractal of solar cycles
Obviously, there was a phase reversal in
1968 that affected the relationship with ENSO events. What happened at
that time? Fortunately, I can give a precise answer based on publications
since 1976 [20-31].
There is an aggregation of solar-terrestrial cycles which are all related
to the sunís fundamental oscillation about the center of mass of the solar
system and form a fractal into which cycles of different length, but similar
function are integrated. The solar dynamo theory, developed by H. Babcock,
starts from the premise that the dynamics of the magnetic sunspot cycle
is driven by the sunís rotation. Yet this theory only takes into account
the sunís spin momentum, related to its rotation on its axis, not its orbital
angular momentum linked to its very irregular motion about the center of
mass. The contribution of the sunís orbital angular momentum to its total
angular momentum is not negligible. It reaches 25 percent of the spin momentum.
The orbital angular momentum can increase or decrease forty-fold within
a few years. Thus it is conceivable that these variations are related to
varying phenomena in the sunís activity. Variations of more than 7 percent
in the sunís equatorial rotational velocity, going along with variations
in solar activity, were observed at irregular intervals. This could be
explained by transfer of angular momentum from the sunís orbit to the spin
on its axis. Part of the necessary spin-orbit coupling could result from
the sunís motion through its own ejected plasma. As R. H. Dicke [10]
has shown, the low corona can act as a brake on the sunís surface.
The four giant planets, which regulate the sunís motion, carry more than
99 percent of the angular momentum in the solar system, while the sun is
confined to less than 1 percent. So there is enough angular momentum that
can be transferred from the outer planets to the revolving sun and eventually
to the spinning sun.
I have shown that cycles derived from the
sunís motion about the center of mass make it possible to predict different
facets of solar activity, including solar eruptions, and climate phenomena
like temperature anomalies, droughts, and El Niños. Observation
shows that the aggregate of physically and structurally connected cycles
forms a hierarchy such that cycles higher up in the hierarchy do not only
modulate subordinated cycles, but also induce phase reversals. Experimentation
with electrical and mechanical control equipment shows that at nodal points,
where the response of the system is zero, the phase can shift by pi radians
[6]. If a hierarchically
dominant cycle reaches a zero phase, which is a nodal point, and a subordinated
cycle is close to an initial phase at the same time, a phase reversal effect
occurs in most cases in the dominated cycle. This seems to solve the problem
of sudden phase jumps in solar-terrestrial cycles hitherto unexplainable
and unpredictable. As to a string of examples I refer to my paper "Solar
Activity: A Dominant Factor in Climate Dynamics" [31]
and other publications [27-31].
The solar cycles in question display five-fold
symmetry and resemble hands and fingers when plotted. So I call them big
hand cycle (178.8 years), big finger cycle (mean length 35.76 years), and
small finger cycle (mean length 7.15 years). Details are given in my paper
quoted above. The acronym BFS in Figure 4 refers to the starting phase
of a big finger cycle. As the 11-year sunspot cycle is shorter than the
big finger cycle, it ranges lower in the hierarchy of solar cycles and
is exposed to the risk of a phase reversal. This all the more so, as the
polar photospheric magnetic fields, linked to the sunís general magnetic
field, underwent a polarity reversal just in 1968, the epoch of the big
finger start in question. In addition, the initial phase of the big finger
cycle coincided with the sunspot maximum 1968, the zero phase of the declining
subcycle from maximum to minimum.
Though I was acquainted with phase reversals
within the fractal of cycles created by the sunís oscillation about the
center of mass, I had not expected that such events would also affect the
11-year sunspot cycle. Yet short reflection shows that it is the same sun
that produces both kinds of cycles. And there is a further link. The magnetic
cycle of 22.1 years, called Hale cycle, is the true cycle of sunspot activity.
Groups of sunspots are usually composed of preceding and following spots
with different magnetic polarity. With the commencement of a new cycle
the polarity reverses so that the original polarity is only restored every
second 11-year sunspot cycle. When the major of the Golden section within
a big finger cycle is calculated, it turns out to be identical with the
length of the Hale cycle (35.76 yrs * 0.618
= 22.1 yrs). This points to a close connection between the big finger cycle
and the Hale cycle as well as the 11-year cycles that compose it.
The circumstance that the Golden section
phases 0.618 and 0.146, which also stand out in Figure 3, do not emerge
in the detailed investigation covering the SOI 1951 - 1998, does not mean
that they are generally not valid. It could be that they are prominent
in other intervals of the span of nearly four hundred years. In 1933, for
instance, the big hand cycle reached a zero phase that could have affected
the effects of the big finger cycle and eventually the subcycles of the
sunspot cycle. It is imaginable that in such cases a switch occurs which
exchanges the pair 0.382/0.854 for 0.146/0.618. Detailed analyses covering
earlier periods are necessary to answer these questions
 7.
Distribution of El Niños within the small finger cycle since 1610
7.
Distribution of El Niños within the small finger cycle since 1610
My correct forecast of the last two El Niños
was based on an analysis of tropical seasurface temperatures in relation
to the small finger cycle (SFC) covering the period 1960 to 1990. The analysis
indicated that the initial phase of the SFC and the major 0.618 within
this cycle are associated with El Niño events. When the cycle was
longer than 7.5 years, also the minor 0.382 proved effective. A further
investigation making use of the El Niño data going back to 1610
should confirm this result, if it is real. The evaluation of the small
finger phases is no problem. They can be calculated back or ahead for thousands
of years. Figure 5 shows the resultant distribution.

The initial phase of the SFC, the minor 0.382,
and the major 0.618 stand out. A chi-square test yields the value 15.2
for 1 degree of freedom. The null hypothesis of no correlation between
the studied variables can be rejected at a high level of significance:
P = 0.0001. As with the sunspot cycle, additional Golden section phases
of the second generation emerge in Figure 5. The phase 0.236 falls at the
major of the interval between 0 and 0.382, and the phase 0.764 is identical
with the minor of the interval between 0.618 and 1. Again, the overall
distribution of the four Golden section phases is symmetrical about the
middle 0.5 of the unit cycle. A chi-square test of all outstanding phases
with ranges of accumulation as indicated in Figure 5 by horizontal bars
yields the chi-square value 34.7 for 1 degree of freedom. The probability
of a chance distribution is P = 4×10-9.
The hypothesis formulated at the outset does not cover the two additional
phases that emerged unexpectedly, but the result deviates so far from the
expected distribution that it points to a real connection.
 8.
Distribution of El Niños within the small finger cycle since 1951
8.
Distribution of El Niños within the small finger cycle since 1951

Figure 6 shows how the SFC phases
0.382 and 0.618, prominent in Figure 5, are in detail associated with SOI
extrema in the interval 1951 - 1998. After BFS 1968 they are closely correlated
with negative extrema (El Niños) and before 1968 with positive extrema
(La Niñas). Initial phases of special small finger cycles (SFS g)
are also indicated, but only tentatively and only after 1968. Before 1968
they do not show the usual reversed pattern, or better, there is no consistent
pattern at all. This speaks against the dependability of this factor, at
least in the period 1951 - 1998. This all the more so as the two cases
where there is a coincidence with El Niños can as well be explained
by 0.382 a,d within subcycles of the sunspot cycle.
Figure 7 displays the detailed relationship
between the two other SFC phases 0.764 (yellow) and 0.236 (blue), outstanding
in Figure 5, and the SOI 1951 - 1998.
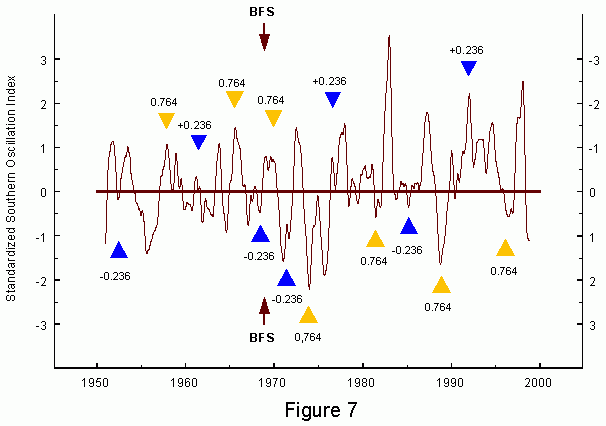
Before BFS 1968, the 0.764 phases are closely
correlated with negative extrema (El Niños) and after 1968 with
positive extrema (La Niñas). The distribution of the SFC phase 0.236
is again a surprise. It displays an alternating pattern, first an association
with El Niño (+ 0.236), then with La Niña (- 0.236), again
with El Niño and so on. BFS 1968 induced a phase reversal such that
another association with La Niña occurred instead of the expected
alternating association with El Niño. I observed such alternating
patterns already in other relationships between cycles derived from the
sunís oscillations about the center of mass and climate phenomena [31].
Such unexpected patterns show as well as the observed phase reversals that
Nature seems to be much more inclined to vary, jump and permute than we
expect. Our result teaches us that crude statistical investigations like
my examination of four centuries of data can give some hints, but cannot
replace a thorough, unprejudiced analysis that elaborates the implications
of a significant correlation. Rigid dogmatism will surely prevent us from
adjusting our world view to Natureís flexibility.
 9.
Synopsis of the correlation between solar cycles and ENSO events
9.
Synopsis of the correlation between solar cycles and ENSO events
Figure 8 shows the synopsis of the
relationship between Golden section phases within the investigated cycles
and SOI extrema phase-locked with El Niños and La Niñas.

Yellow circles mark correlations of phases
within the subcycles of the 11-year sunspot cycle with El Niños
and blue circles with La Niñas. Bright green diamonds point to connections
of SFC phases with El Niños and dark green diamonds with La Niñas.
It is easy to see that all notable deviations from the zero line are explained
with the exception of a single El Niño at the beginning of the curve.
It should be noted that all of the respective Golden section phases that
fall into the period 1951 - 1998 appear in the synopsis. There is not a
single one that does not coincide with SOI extrema. Because of the phase
reversal induced by BFS 1968 the same phases can be linked to El Niños
as well as to La Niñas and can be used to predict both of these
events depending on the phase of the dominating big finger cycle. There
are no exceptions to this consistent pattern. Climatologists have been
wondering why there were three consecutive El Niños without any
interruption by La Niñas between 1991 and 1995. Figure 8 gives the
answer. During the five years in question there were not any Golden section
phases that indicate La Niñas, but four of them that point to El
Niños.
 10.
Maximum entropy spectral analysis of SOI data 1951 - 1998
10.
Maximum entropy spectral analysis of SOI data 1951 - 1998
These results are confirmed by a different
approach. J. P. Burg [5]
has developed a new form of spectral variance analysis, the maximum
entropy spectral method which shows much higher resolution than earlier
frequency analyses, especially at lower frequencies. Figure 9 shows
the maximum entropy spectrum of the SOI data 1951 - 1998.
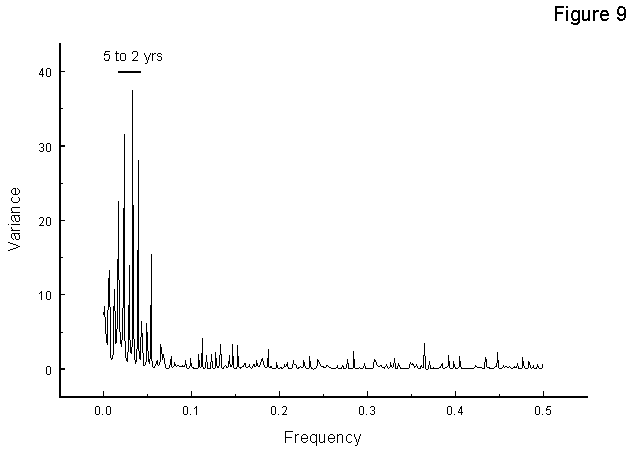
The frequency is measured in cycles per month.
The analysis is based on 575 data points and makes use of a filter length
of 230, which is in accordance with the suggestion of most authors not
to go beyond 40 percent of the length of the time series to avoid spectral
shifts. The spectrum shows prominent peaks only in the frequency range
0 to 0.06. The four outstanding peaks lie in the period range between 2
and 5 years. The resolution is much finer than in the inset in Figure 1.
Figure 10 sets off the frequency range
of interest between 0 and 0.1 and shows an even better resolution.
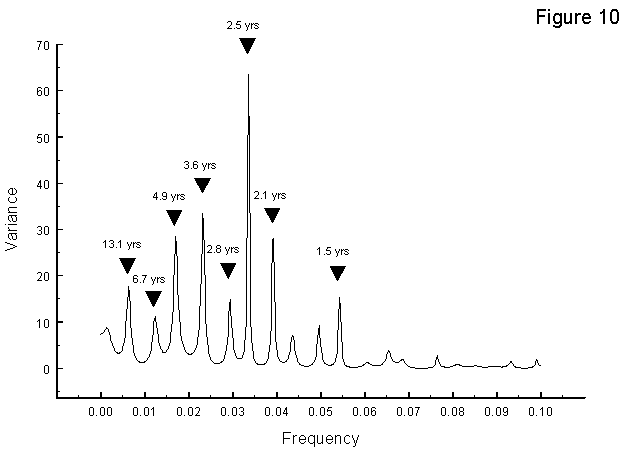
The periods of the prominent peaks are indicated.
They can be compared with the distance of the crucial Golden section phases
from the zero phases of the investigated three cycles. In the course of
the years 1951 - 1998 these cycles had the following mean lengths: the
ascending subcycle (a) of the sunspot cycle (SC) 3.4 years, the declining
subcycle (b) 7.1 years, and the small finger cycle (SFC) 6.8 years. In
the following, the distances of the Golden section phases from the beginning
of the respective cycle are given first and then in parantheses the periods
of the maximum entropy spectrum (MES) that come closest:
| SC 0.382 a: |
1.3 years |
(MES: 1.5 years) |
| SFC 0.236: |
1.6 years |
(MES: 1.5 years) |
| SFC 0.382: |
2.6 years |
(MES: 2.5 and 2.1 years) |
| SC 0.382 d: |
2.7 years |
(MES: 2.8 and 2.5 years) |
| SFC 0.618: |
4.2 years |
(MES: 3.6 years) |
| SFC 0.764: |
5.2 years |
(MES: 4.9 years) |
| SC 0.854 d: |
6.1 years |
(MES: 6.7 years) |
Considering the uncertainties inherent in
spectrum analysis, especially at low frequencies, this is a rather exact
match. It is corroborated by comparing the means of the two data sets.
The mean of the distances of the seven Golden section phases from the beginning
of the respective cycles is 3.4 years. The mean of the outstanding SOI
periods, marked in Figure 10, is also just 3.4 years when we exclude the
long period of 13.1 years that is far beyond the range which is of interest
in this respect. It should be noted, however, that 13.1 is close to the
second subharmonic of the MES period 6.7 years. The long period could be
of import with respect to the strength of ENSO events. The interval between
the two very strong El Niños 1982/1983 and 1987/1988 is relatively
close to that period. As to modulations of the strength of El Niños
it is of interest, too, that the SC and SFC periods 1.3 years and 1.6 years
as well as 2.6 years and 2.7 years are so close to each other that resonance
is possible.
Finally, it should be noted that the highest
spectral peak at 2.5 years falls at the phase 0.382 of the descending sunspot
cycle that stands out in every respect and is together the phase of the
outstanding accumulation of energetic solar eruptions in Figure 2. The
prominent periods of the maximum entropy spectrum are significant. An acknowledged
reliability test of maximum entropy peaks does not exist, but according
to a simple and useful rule of thumb, given by W. F. Stuart, V. Sherwood,
and S. M. MacIntosh [50],
a spectral peak is regarded to be significant if it contains at least three
computed points which deviate from the noise and has a maximum two or three
times greater than the surrounding noise level. On the basis of this standard
the peaks marked in Figure 10 deviate significantly from the noise.
A further confirmation is provided by a Blackman-Tukey
power spectrum [3]
of the SOI data 1951 - 1998. The four outstanding peaks of this spectrum
at 2.1, 2.6, 3.8, and 4.8 years nearly coincide with the respective maximum
entropy peaks, and the mean of these four periods equals 3.3 years, rather
close to the maximum entropy mean of 3.4 years. H. A. Panofsky and G. W.
Brier [39] have
developed a special reliability test of peaks in a Blackman-Tukey spectrum.
Checked against a white noise background, the peaks in Figure 10 go far
beyond the 99 percent confidence level. The significance of the deviation
from the Markov red noise level, taking into account potential autocorrelation,
reaches at least the 95 percent level.
 11.
Forecasts of future ENSO events
11.
Forecasts of future ENSO events
Precise forecasts that prove correct are
the sharpest criterion of effective science. So I will try my third long-range
El Niño forecast. It goes nearly three years beyond the lead time
of 12 months, discussed in the beginning. The next negative extremum
in the SOI going along with an El Niño should occur around 2002.9
(± 6 months). The last zero phase of a small finger cycle fell
at 1998.3. The following one will occur in 2005.8. The length of the SFC
cycle reaches just 7.5 years. So the probability is low, though not zero,
that the phase 0.382 within the SFC will release an El Niño around
2001.2. The following 0.618 phase falls at 2002.9. It should be effective.
This all the more so as around this time the descending part of the sunspot
cycle is expected to reach the phase 0.382. The next negative 0.236 phase
within the small finger cycle will fall at 2000.1. The present positive
SOI and the accompanying La Niña seem to be related to it. So La
Niña conditions should prevail till 2000.1 and beyond.
 12.
Objections to a strong link between solar activity and climate
12.
Objections to a strong link between solar activity and climate
Taken together, the lines of evidence presented
here leave little doubt that the relationship between Golden section phases
within solar cycles and ENSO events is real. Nonetheless, it is to be expected
that sceptics will point at the lack of detailed cause and effect arguments
and properly quantified physical mechanisms. Seen in a historical light,
such objections are not valid. The lack of elaborate theory does not impair
the heuristic importance of the results.You cannot achieve everything at
the same time. Epistemologically, the stage of gathering data, establishing
morphological relations, and setting up working hypotheses necessarily
precedes the stage of elaborated theories. How can we solidly connect solar
activity with climatic change as long as neither of these fields rests
on a solid theoretical foundation? An accepted full theory of solar activity
does not yet exist. What we have is only the hope of a future theory. According
to P. V. Foukal [11]
the mechanism that causes the solar magnetic cycle remains poorly understood,
although it has been the focus of intense research during the past half
century. There is a lot of literature about aw-dynamos,
but they are coping with incompatibilities of observation with theory,
and they do not offer any explanation of longer solar cycles like the Gleissberg
cycle that modulates the amplitudes of the 11-year cycle.
The understanding of climate change, too,
is in a rudimentary stage. Especially complex coupling processes that could
link the upper with the lower atmosphere are far from being well understood,
and even the most advanced general circulation models yield contradictory
results. Revealing is a recent statement by J. E. Hansen [13],
a protagonist of global warming:
"The forcings
that drive long-term climate change are not known with an accuracy sufficient
to define future climate change ... The natural forcing due to solar irradiance
changes may play a larger role in long-term climate change than inferred
from comparisons with general circulation models alone."
Hansen does not even mention the effect of
solar eruptions and the solar wind on climate.
The usual additional objection that the solar
effect is much too weak to affect climate, too, is not tenable. J. G. Roederer
[47] has aptly remarked:
"The energy
argument is not valid for highly nonlinear complex systems such as the
coupled atmosphere-ocean-cryosphere-biosphere. It is well known that complex
systems can behave chaotically, i.e., follow very different paths after
the smallest change in initial or boundary conditions, or in response to
the smallest perturbation. In a highly nonlinear system with large reservoirs
of latent energy such as the atmosphere-ocean-biosphere, global redistributions
of energy can be triggered by very small inputs, a process that depends
far more on their spatial and temporal pattern than on their magnitude."
H. Svensmark and E. Friis-Christensen
[53] have demonstrated
that this is in accordance with reality. Clouds have a hundred times stronger
effect on weather and climate than carbon dioxide in the atmosphere. Even
if the atmosphereís carbon dioxide content doubled, its effect would be
cancelled out if the cloud cover expanded by 1 percent, as shown by H.
E. Landsberg [19].
So it is of great importance that Svensmark and Friis-Christensen have
shown that global cloud cover, observed by satellites, is linked to the
strength of cosmic rays modulated by the solar wind. When the solar wind
is strong and cosmic rays are weak, the global cloud cover shrinks. It
extends when cosmic rays are strong because the solar wind is weak. This
effect, attributed to cloud seeding by ionized secondary particles, causes
a change in cloud cover by more than 3 percent within 3 ½ years.
The corresponding change in solar irradiance reaches about 1.5 W/m2.
This is a considerable amount, since the total radiative forcing by carbon
dioxide accumulated in the atmosphere since 1750 does not go beyond 1.5
W/m2. One would assume that such a tremendous
effect should be caused by a huge amount of energy, but cosmic rays inject
a total energy into the atmosphere that is very small. Astonishingly, it
is equal to the intensity of starlight in the night skies [16].
This is practical proof that Roedererís argument is not only of theoretical
import.
 13.
Potential connections between solar eruptions and ENSO events
13.
Potential connections between solar eruptions and ENSO events
Though there are no strict physical arguments
that could explain in detail how solar activity causes ENSO events, it
is quite possible to develop working hypotheses that suggest potential
connections. Figure 2 shows that energetic solar eruptions coincide with
the Golden section phase 0.382 in the subcycles of the sunspot cycle which
are closely correlated with ENSO events, as shown in Figure 4. Strong solar
eruptions cause the highest velocities in the solar wind and create shockwaves
that compress and intensify magnetic fields in the sunís plasma moving
outward to the boundary of the solar system. The solar wind strenghtened
by solar eruptions weakens cosmic rays. The ensuing Svensmark-effect is
regionally strongest where cloudiness is highest. It is very high around
Indonesia [40] where
el Niños seem to develop. So one would think that shrinking cloud
cover, stronger irradiance, intensified Hadley circulation and changing
trade winds, caused by the modulating effect of solar eruptions on cosmic
rays, improve the conditions for the birth of El Niños. This all
the more so as M. Pudvokin and S. Veretenenko [42]
as well as Svensmark and Friis-Christensen [53]
have shown that Forbush decreases - dips in cosmic rays by several
percent within 2 days after a strong solar eruption - are associated with
immediate decreases in cloudiness by 2 - 3 percent that last a week or
longer. Such short-term effects, especially when they trigger tropical
cyclones [44],
may release and sustain El Niños.
The enhancement of the Svensmark-effect by
the very high cloudiness around Indonesia is countered by the circumstance
that Indonesia is situated on the equator where the field lines of the
Earthís magnetic field run parallel with the surface. As less energetic
cosmic-ray particles follow the magnetic field lines on screw shaped trajectories,
it is more difficult for them to penetrate into the atmosphere above the
equator than near the poles where the field lines run vertically. This
magnetic obstacle is especially effective when particles try to penetrate
to the Earthís surface. They reach the ground only when their energy is
at the 15 GeV level. Yet it is much easier to reach targets higher up in
the atmosphere. One of the stations that observe cosmic rays, the Huancayo
Neutron Monitor, is located close to the equator (12° S 75° W),
but at an altitude of 3400 m where the cutoff rigidity is not as high.
The Huancayo reports reflect the change in cosmic rays as well as data
by other stations at higher latitudes. Especially, it has to be taken into
consideration that high clouds like cirrus, cirrocumulus, and cirrostratus
reach altitudes of 18 km at the equator where the height of the tropopause
goes far beyond that at the poles (8 km). Cumulonimbus reaching an altitude
of 10 - 14 km at temperate latitudes climb to 16 - 22 km in the tropics,
where they form huge cloud clusters covering ranges of more than 100 km
[33]. The generation
of secondary cosmic rays by primary cosmic rays and the related degree
of ionization reach a maximum (300 pairs of ions/cm3 sec) just at 20 km
altitude [16],
close to the altitude of tropical cumulonimbus as well as of cirrus, cirrocumulus,
and cirrostratus.
Furthermore, the geomagnetic equator, not
the geographical equator is relevant as to the cutoff conditions of cosmic
ray particles. At present the observed geomagnetic pole in the Northern
Hemisphere is at 73° N 100 W. Calculation shows that a location on
the equator in Indonesia (longitude 105° E) has a geomagnetic latitude
of -15° where particles can easier penetrate to those high altitudes
where clouds are to be found in the tropics. All arguments taken together,
it is not unimaginable that the Svensmark-effect works in the region close
to the equator where El-Niños are thought to come into existence.
Conditions should be favourable for La Niña when cosmic rays are
very strong because the sunís eruptional activity is exceptionally weak.
Further working hypotheses may be based on
the fact that solar X-rays and UV radiation increase sharply at the time
of energetic solar eruptions. It would go beyond the frame of this paper
to describe these working hypotheses in detail. They can only be presented
in a nutshell. Flares increase the sunís UV radiation level by at least16
percent [15].
Ozone in the stratosphere absorbs this excess energy that causes local
warming. The 70-mb polar vortex is displaced. This disturbance is propagated
downward to the troposphere where it affects the intensity of the Hadley
circulation. D. E. Hartley, J. T. Villarin, R. X. Black , and C. A. Davis
[14] have shown that there is a dynamical link between
stratospheric polar vortex distortions and meteorological events in the
troposphere. Observations by other authors confirm this result [12,
18, 37, 45, 46]. As El Niños are linked to trade
winds and tradewinds to the Hadley cells that may be affected by circulation
change in the stratosphere, it seems plausible that energetic solar eruptions
could be the cause of this chain of links. This all the more so as observations
show distinct change in diverse weather phenomena within days after energetic
solar eruptions [4, 8, 48, 49, 55].
Solar X-rays around 10 Å intensify
by a factor of 100 or more during moderate-sized flares, and strong flares
can amplify the X-ray level by a factor of 1000. I refer to my paper "Solar
Rotation, Impulses of the Torque in the Sunís Motion, and Climatic Variation"
[25] which describes
how strong X-rays produced by energetic solar eruptions may enhance thunderstorm
activity. Severe thunderstorms are linked to tropical cyclones [54]
which may trigger and sustain El Niños [44].
A marked lull in the sunís UV radiation and X-rays should be favourable
for La Niñas.
These theoretical arguments were only presented
to show that it is not out of the question that there are physical links
between energetic solar eruptions and El Niños. Whether these lines
of reasoning turn out correct or spurious is of no import regarding the
practical results of this investigation. They leave little doubt that solar
activity and ENSO events are closely connected to such a degree that long-range
forecasts beyond the 12-month lead time are now possible. The consequences
of these results for the hypothesis of anthropogenic climate change are
far-reaching. As stated in the beginning, ENSO events are the strongest
source of variability in the global climate system and explain most of
the global temperature anomalies. Our result that solar activity regulates
these powerful climate phenomena shows clearly that the impact of the sunís
variability has been underestimated in a way that reverses the proportions.
Recent research published by H. Svensmark [52]
and N.
Calder [7] corroborate
this statement. Actually, solar activity turns out to be the dominant factor
in climate change. IPCC scientists can no longer uphold their contention
that "solar variability over the next 50
years will not induce a prolonged forcing significant in comparison with
the effect of increasing carbon dioxide concentrations."

 Acknowledgements
Acknowledgements
I owe special thanks to Nigel Calder for
his suggestion to investigate associations between El Niños and
special phases in the 11-year sunspot cycle. I am a convert in this respect.
Before Nigel Calderís stimulating intervention I thought that only cycles
based on the sunís motion about the center of mass of the solar system
could affect ENSO events.
 References
References
[1] Arnolíd, V. I.: Small denominators
and problems of stability of motion in classical and celestial mechanics.
Russ. Math. Surv. 18 (1963), 85.
[2] Arntz, W. A. and Fahrbach,
E.: El Niño. Klimaexperimente der Natur. Basel, Birkhäuser,
1991.
[3] Blackman, R. B. and Tukey,
J. W.: The measurement of power spectra. New York, Dover, 1959.
[4] Bossolasco, M., Dagnino,
I., Elena, A., and Flocchini, G.: Thunderstorm activity and interplanetary
magnetic field. Riv. Italiana Geofis. 22 (1973), 293.
[5] Burg, J. P.: Maximum entropy
analysis. Ph.D. thesis. Stanford University, Palo Alto, 1975.
[6] Burroughs, W. J.: Weather
cycles - real or imaginary? Cambridge University Press,1992, 149.
[7] Calder, N.: The carbon
dioxide thermometer and the cause of global warming. http://www.john-daly.com/calder/calder.htm.
[8] Cobb, W. E.: Evidence of
solar influence on the atmospheric electric elements at Mauna Loa Observatory.
Mon. Weather Rev. 95 (1967), 905-911.
[9] Daly, J. L.: El Niño
and global temperature. http://www.john-daly.com/soi-temp.htm.
[10] Dicke, R. H.: The sunís
rotation and relativity. Nature 202 (1964), 432.
[11] Foukal, P. V.: The variable
sun. Scient. American, Februar 1990, 34-41.
[12] Haigh, J. D.: The impact
of solar variability on climate. Science 272 (1996), 981-984.
[13] Hansen, J. E., Sato, M.,
Lacis, A., Ruedy, R., Tegen, I., and Matthews, E.: Climate forcings in
the industrial era. Proceedings of the National Academy of Sciences 95
(1998), 12753-12758.
[14] Hartley, D. E., Villarin,
J. T., Black, R. X., and Davis, C. A.: A new perspective on the dynamical
link between the stratosphere and troposphere. Nature 391 (1998), 471-474.
[15] Heath, D.: The ultraviolet
region. In: White, O. R.: The solar output and its variation. Boulder,
Colorado Associated University Press, 1977, 32.
[16] Kertz, W.: Einführung
in die Geophysik II. Mannheim, Bibliographisches Institut, 1971, 340, 341.
[17] Kolmogorov, A. N.: Preservation
of conditionally periodic movements with small change in the Hamiltonian
function. Lecture Notes in Physics 93 (1979), 51.
[18] Labitzke, K. und van Loon,
H: Some recent studies of probable connection between solar and atmospheric
variability. Ann. Geophysicae 11 (1993), 1084.
[19] Landsberg, H. E.: Man-made
climatic changes. In: Proceedings of the symposium on physical and dynamic
climatology of the World Meteorological Organization 347 (1974), 262.
[20] Landscheidt, T.: Beziehungen
zwischen der Sonnenaktivität und dem Massenzentrum des Sonnensystems.
Nachrichten der Olbers-Gesellschaft 100 (1976).
[21] Landscheidt, T.: Solar
oscillations, sunspot cycles, and climatic change. In: McCormac, B. M.,
ed.: Weather and climate responses to solar variations. Boulder, Associated
University Press, 1983, 293-308.
[22] Landscheidt, T.: Long-range
forecast of energetic x-ray bursts based on cycles of flares. In: Simon,
P. A., Heckman, G. und Shea, M. A., eds.: Solar-terretrial predictions.
Proceedings of a workshop at Meudon, 18.-22. Juni 1984. Boulder, National
Oceanic and Atmospheric Administration, 1986, 81-89.
[23] Landscheidt, T.: Long-range
forecast of sunspot cycles. In: Simon, P. A., Heckman, G. und Shea, M.
A., eds.: Solar-terretrial predictions. Proceedings of a workshop at Meudon,
18.-22. Juni 1984. Boulder, National Oceanic and Atmospheric Administration,
1986, 48-57.
[24] Landscheidt, T.: Long-range
forecasts of solar cycles and climate change. In: Rampino, M. R., Sanders,
J. E., Newman, W. S. und Königsson, L. K.,eds.: Climate. History,
Periodicity, and predictability. New York, van Nostrand Reinhold, 1987,
421-445.
[25] Landscheidt, T.: Solar
rotation, impulses of the torque in the sunís motion, and climatic variation.
Climatic Change 12 (1988), 265-295.
[26] Landscheidt, T.: Relationship
between rainfall in the northern hemisphere and impulses of the torque
in the sunís motion. In: K. H. Schatten und A. Arking, eds.: Climate impact
of solar variability. Greenbelt, NASA, 1990, 259-266.
[27] Landscheidt, T.: Global
warming or Little Ice Age? In: Finkl, C. W., ed.: Holocene cycles. A Jubilee
volume in celebration of the 80th birthday of Rhodes W. Fairbridge. Fort
Lauderdale, The Coastal Education and Research Foundation (CERF), 1995,
371-382.
[28] Landscheidt, T.: Die kosmische
Funktion des Goldenen Schnitts. In: P. H. Richter, Hsg.: Sterne, Mond und
Kometen. Bremen und die Astronomie. Bremen, Verlag H. M. Hauschild, 1995,
240-276.
[29] Landscheidt, T.: Klimavorhersage
mit astronomischen Mitteln? Nachrichten der Olbers-Gesellschaft Bremen,
July 1997, 10-14, October 1997, 4-8.
[30] Landscheidt, T.: Forecast
of global temperature, El Niño, and cloud coverage by astronomical
means. In:Bate, R., ed.: Global Warming. The continuing debate. Cambridge,
The European Science and Environment Forum
(ESEF), 1998, 172-183.
[31] Landscheidt, T.: Solar
activity: A dominant factor in climate dynamics. Energy and Environment,
in print. http://www.john-daly.com/solar/solar.htm.
[32] Laskar, J.: A numerical
experiment on the chaotic behaviour of the solar system. Nature 338 (1989),
237.
[33] Möller, F.: Einführung
in die Meteorologie I, Mannheim, B.I.-Wissenschaftsverlag, 1984, 192.
[34] Moser, J.: Stable and
random motions in dynamical systems. Princeton University Press, 1973.
[35] National Geophysical Data
Center, Boulder: X-ray flares. SOLRAD (1968-1974), GOES (1975-present).
[36] Neelin, J. D. and Latif,
Mojib: El Niño dynamics. Physics Today, December 1998, 32-36.
[37] Neubauer, L.: The sun-weather
connection. Sudden stratospheric warmings correlated with sudden commencements
and solar proton events. In: McCormac, B. M., ed.: Weather and climate
responses to solar variations. Boulder, Colorado Associated University
Press, 1983, 395-397.
[38] NOAA, Climate Prediction
Center, Data and Indices: http://nic.fb4.noaa.gov.
[39] Panofsky, H. A. and Brier,
G. W.: Some applications of statistics on meteorology. The Pennsylvania
State University, 1958.
[40] Peixoto, J. P. und Oort,
A. H.: Physics of climate. New York, American Institute of Physics, 1992,
173, 415, 423, 426, 430.
[41] Philander, S. G. H.: El
Niño, La Niña, and the Southern Oscillation. San Diego, Academic
Press, 1990.
[42] Pudvokin, M. and Veretenenko,
S.: Cloudiness decreases associated with Forbush decreases of galactic
cosmic rays. J. Atm. Terr. Phys. 57 (1995), 1349-1355.
[43] Quinn, W. H., Neal, T.
V., and de Mayolo, S. E. A.: El Niño occurrences over the past four
and a half centuries. Journ. of Geophys. Res. 92 (1987), 14449-14461.
[44] Ramage, C. S.: El Niño.
Scient. American, June 1986, 59-61.
[45] Reiter, R.: Influences
of solar activity on the exchange intensity between stratosphere and troposphere.
In: B. M. McCormac and T. A. Seliga, eds.: Solar-terrestrial influences
on weather and climate. Dordrecht, Reidel, 1979, 289-296.
[46] Reiter, R.: Modification
of the stratospheric ozone profile after acute solar events. In: McCormac,
B. M., ed.: Weather and climate responses to solar variations. Boulder,
Colorado Associated University Press, 1983, 95-116.
[47] Roederer, J. G.: Solar
variability effects on climate. In: B. Frenzel, Hsg.: Solar output and
climate during the Holocene. Stuttgart, Gustav Fischer Verlag, 1995, 3,
17.
[48] Schuurmans, C. J. E.:
Effects of solar flares on the atmospheric circulation. In: McCormac, B.
M. and Seliga, T. A., eds.: Solar-terrestrial influences on weather and
climate. Dordrecht, D. Reidel, 1979, 105-118.
[49] Stolov, H. I. and Shapiro,
R.: Investigation of the responses of the general circulation at 700 mb
to solar geomagnetic disturbance. J. Geophys. Res. 79 (1974), 2161-2170.
[50] Stuart, W. F., Sherwood,
V., and MacIntosh, S. M.: Pure appl. Geophys. 92 (1971), 150.
[51] Sussman, G. J. and Wisdom,
J.: Chaotic evolution of the solar system. Science 257 (1992), 56.
[52] Svensmark, H.: Influence
of cosmic rays on Earthís climate. Physical Review Letters, in print.
[53] Svensmark, H. und Friis-Christensen,
E.: Variation of cosmic ray flux and global cloud coverage, a missing link
in solar-climate relationships. J. Atm. Sol. Terr. Phys. 59 (1997), 1225.
[54] Williams, N. R.: Tornadoes,
whirlwinds, and waterspouts. Encyclopaedia Britannica, Macropaedia, vol.
18, 518.
[55] Wilcox, J. M.: Solar activity
and the weather. J. Atmosph. Terr. Phys. 37 (1975), 237.

In line
with the `Open Review' policy of this website, comments are invited on
the above paper by Dr Theodor Landscheidt and will be published in a separate
file here. All comments,
whether supportive or critical, will be published unedited
(excepting those of a personal or ad hominem
nature).
Comments
should be addressed to daly@microtech.com.au
with "El Nino and the Sun"
in the subject line. -
John L. Daly

Return to Climate
Change Guests Debate
Return to "Still
Waiting For Greenhouse" main page

![]()
![]() 1.
Forecast of ENSO events
1.
Forecast of ENSO events 








